The Lebesgue measure, being a function from family of subsets
![]() of
of ![]() into
into ![]() , is not of the form
, is not of the form
![]() , so it does not lie directly in a scope of this article.
However, it is certainly one of the main tools of real analysis and many
results concerning its generalizations have a deep set theoretical context.
Therefore, a short section concerning
the newest developments in this area has been
added to this paper.
, so it does not lie directly in a scope of this article.
However, it is certainly one of the main tools of real analysis and many
results concerning its generalizations have a deep set theoretical context.
Therefore, a short section concerning
the newest developments in this area has been
added to this paper.
An accessible survey concerning different extensions of Lebesgue
measure can be found in the 1989 Mathematical Intelligencer
article [22] of K. Ciesielski. The best survey concerning
universal (i.e., defined on ![]() ) countable additive extensions of
Lebesgue measure can be found in the 1993 survey article of
D. H. Fremlin [58]. Thus, we will concentrate here only on the newest
developments, that concern isometrically invariant extensions of Lebesgue
measure. (See also M. Laczkovich survey article [90] on this subject.)
) countable additive extensions of
Lebesgue measure can be found in the 1993 survey article of
D. H. Fremlin [58]. Thus, we will concentrate here only on the newest
developments, that concern isometrically invariant extensions of Lebesgue
measure. (See also M. Laczkovich survey article [90] on this subject.)
Recall here, that by the 1923 theorem of Banach there is a finitely additive
isometrically invariant measure ![]() extending
Lebesgue measure, while such a measure on
extending
Lebesgue measure, while such a measure on ![]() does not exist by a famous
Banach-Tarski Paradox (1924):
does not exist by a famous
Banach-Tarski Paradox (1924):
The second famous question was the Tarski's circle-squaring problem: is a
circle ![]() of the unit area equivalent to a square
of the unit area equivalent to a square
![]() of the unit area? Note that if the areas of C and D were
different, then Banach's theorem of 1923 would immediately imply the
negative answer. However, the answer to Tarski's circle-squaring problem is
positive, as proved by Laczkovich in 1990.
of the unit area? Note that if the areas of C and D were
different, then Banach's theorem of 1923 would immediately imply the
negative answer. However, the answer to Tarski's circle-squaring problem is
positive, as proved by Laczkovich in 1990.
The other class of isometrically invariant extensions of Lebesgue measures on
![]() concerns countably additive extensions. In 1936 Sierpinski asked,
whether such an extension can be maximal. The negative answer to this question
was given in 1977 by A. B. Kharazishvili [77] (for n=1) and in 1985 by
Ciesielski and Pelc [40] (for arbitrary n). (Compare also
[23, 24, 85, 143].)
concerns countably additive extensions. In 1936 Sierpinski asked,
whether such an extension can be maximal. The negative answer to this question
was given in 1977 by A. B. Kharazishvili [77] (for n=1) and in 1985 by
Ciesielski and Pelc [40] (for arbitrary n). (Compare also
[23, 24, 85, 143].)
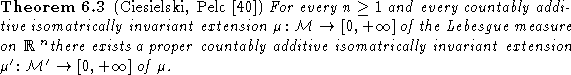
A weak side of this theorem was that the extension ![]() of
of
![]() was only by (new) measure zero sets so, in a way, trivial. This has been
recently improved by Zakrzewski, who showed
was only by (new) measure zero sets so, in a way, trivial. This has been
recently improved by Zakrzewski, who showed
Many interesting results concerning isometrically invariant extensions of Lebesgue measure can be also found in 1983 book of Kharazishvili [78].